Andart

Hospital Art

Laura Splan (via mindhacks) does medical art, artworks inspired by medicine but also using its materials - surgical instruments, blood and instructions.

I was particularly taken by the blood scarf, where a scarf made of clear vinyl tubing drains blood from the arm, becoming a cheerful red and warm scarf that at the same time vampirizes the wearer. Other highlights are the meticulous neuroanatomy drawings done with the artist's blood, doilies based on viruses and the comforting Thorazine pillow.

As more and more of our money, time and culture starts to orbit health and medicine it makes sense to have more medical art. There is certainly no shortage of art depicting illness, pain or being alienated in the medical system. But what about the art that celebrates, plays with or inquires into it? That is harder, because it requires more knowledge and contact with it.

Achille Ghidoni, a professor of genetics, has done some excellent surreal pictures based on genetics and surgery. I especially like his evolution images, suggesting a kind of transbiological future.

Bodyworlds is another obvious example. Although it may have become the Las Vegas tour of anatomy, it does have some very impressive pieces. The questions posed by the exhibition about the separation - and desirability of separating - art and medicine are fun.

Then there is of course the entire realm of biotech art, although it is usually much more conceptual like Eduardo Kac's "Move 36" and the tissue culture and art project. Fun, but not quite medicine yet.

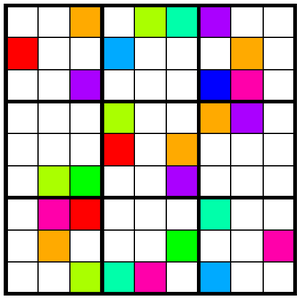
Color sudoku

Here is a little demonstration of how our affordances can make formally identical tasks differently hard.

I took a sudoku puzzle from Daily SuDoku and replaced the numbers with colors. It works just as normal, you have to fill in the colors so that each color occurs within each 3x3 matrix and along each row and column of the larger square. The simplest way of trying it may be to download the picture into a paint program and use the fill tool.

Easy, isn't it?

Colors do not have a discrete representation in our visual system unless occuring in a small simple set such as red-green-yellow-blue. Hence our ability to easily recognize whether there is a duplication or a gap in a color set that doesn't fit in with this will be impaired. While numbers are easily handled as discrete objects, perhaps through a spatial or verbal representation. Several sites have sudoku puzzles that show numbers with colors, which presumably makes things easier by enabling different minds to use whatever strategies fit them.

There is a color sudoku game out there, but it seems to start with colors selected for maximal discreteness (my set is equidistant by 40 degrees along the color circle instead).

The Flickr sudoku game seems to bear this out. Here the elements are images, again with no easy set membership detection. Of course, having to do saccades all the time to the list slows things down too.

Knotted minimal surfaces

All knots can be spanned by an oriented nonsingular minimal surface, and my particular favorite is the threefoil knot surface. While playing around with surface evolver I got the idea of attaching a catenoid handle to it, producing this rather neat surface.

An obvious generalisation is to add a catenoid to the top too, producing a three-ended minimal surface.

I wonder how the knotted end of the surface continues. It seems rather likely that it will self-intersect and behave badly, although the "blades" look somewhat helicoidal. I don't know any reliable method of extending a meshed minimal surface onwards (lots of numerical instability issues).

Here is a rendering of the surface.

ADDENDUM: An obvious extension is to splice two knot ends together using the catenoid. The result is a nicely messy surface. There are of course two variants, depending on the parity of the knots. The width of the neck between them depends on their distance like in an ordinary catenoid; beyond a certain point the minmal surface pinches off.

Here is a rendering of a fairly "thick-necked" version of the surface.

Googling for health

This weeks post on CNE health was about using google for diagnosis. The funniest part about the research is not the paper but the comments.

As I have written earlier something utterly new happens when a lot of information is online. Individual snowflakes are utterly different from a glacier. Dedicated search engines look promising, especially if people can put them together themselves.

Genes, Eve and Cheese Mazes

This week's CNE Health blog is about the prospects for biogenerics; looking good thanks to the US democrats and a the grinding EU process, but I still think the Indians are going to be the big winners.

Last week was about making gene names non-upsetting. Personally I think this is a silly issue, but it led me to this site about fun gene names. I think my favorite is Pray for Elves (scroll down to "Other information" for an explanation). Of course the pokemon gene is very useful, as this cartoon demonstrates. And while we are at it, I must join the chorus of praise for redressing the honor of Eve!

Hmm, since this has already become a surreal association game, I might as well mention my latest timewaster, Baron von Puttyngton versus the Cancerous M.C. Escher Maze of Cheese. A magnificient game where you play a pink jelly cube trundling through a growing Escher maze, turning yellow cheese into blue to stop the maze from growing. To do this you can change the direction of gravity. It is marvellous in its surreal simplicity and weirdness. No monsters, no time countdown, just you against geometry, rubberiness and exponential growth.

Minimal Double Walls

Yesterday when playing around with the Enneper-Weierstrass formula for minimal surfaces. I plugged in g(z)=tanh(z) and f(z)=1, producing this nice minimal surface.

It is parametrized by [a,b,c]=Re[tanh(z),i*(2z-tanh(z)), 2ln(cosh(z))].

The basic structure is neat and simple. An almost plane surface bends like a book spine, erupting in an infinite number of catenoid flares. These rudely intersect as catenoids are wont to do, preventing embedding. It is topologically equivalent to an infinitely punctured plane due to all the singularities at ±(2n+1)π/2. Each singularity causes a flare from the bending region.

I have not seen this minimal surface before. Trying g(z)=cosh(z) and g(z)=sinh(z) produces what I think is the Henneberg nonorientable surface. But it seems unlikely that something as obvious as tanh(z) could have been overlooked, so I wonder what this surface is called.

One explanation might be that it doesn't have many useful properties: while the planes look inviting for building something they are very gently undulating rather than being perfect planes and hence cannot be mirrored to produce another line of flares. Still, my surface looks like a limiting case of the k-Noid as k goes to infinity.

Drawing it nicely posed a few problems. Just using a rectangular mesh produced jaggedness close to the singularities. In the end I had the idea of using both a rectangular point grid to get the overall plane part nicely triangulated, and adding radial point grids near the singularities. Then I used a Delaunay triangulation to form the base mesh.