The end of the worlds

George Dvorsky has a piece on Io9 about ways we could wreck the solar system, where he cites me in a few places. This is mostly for fun, but I think it links to an important existential risk issue: what conceivable threats have big enough spatial reach to threaten a interplanetary or even star-faring civilization?

This matters, since most existential risks we worry about today (like nuclear war, bioweapons, global ecological/societal crashes) only affect one planet. But if existential risk is the answer to the Fermi question, then the peril has to strike reliably. If it is one of the local ones it has to strike early: a multi-planet civilization is largely immune to the local risks. It will not just be distributed, but it will almost by necessity have fairly self-sufficient habitats that could act as seeds for a new civilization if they survive. Since it is entirely conceivable that we could have invented rockets and spaceflight long before discovering anything odd about uranium or how genetics work it seems unlikely that any of these local risks are “it”. That means that the risks have to be spatially bigger (or, of course, that xrisk is not the answer to the Fermi question).

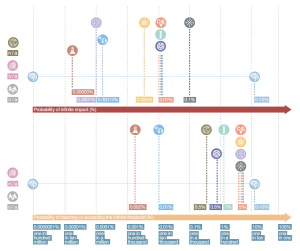
Of the risks mentioned by George physics disasters are intriguing, since they might irradiate solar systems efficiently. But the reliability of them being triggered before interstellar spread seems problematic. Stellar engineering, stellification and orbit manipulation may be issues, but they hardly happen early – lots of time to escape. Warp drives and wormholes are also likely late activities, and do not seem to be reliable as extinctors. These are all still relatively localized: while able to irradiate a largish volume, they are not fine-tuned to cause damage and does not follow fleeing people. Dangers from self-replicating or self-improving machines seems to be a plausible, spatially unbound risk that could pursue (but also problematic for the Fermi question since now the machines are the aliens). Attracting malevolent aliens may actually be a relevant risk: assuming von Neumann probes one can set up global warning systems or “police probes” that maintain whatever rules the original programmers desire, and it is not too hard to imagine ruthless or uncaring systems that could enforce the great silence. Since early civilizations have the chance to spread to enormous volumes given a certain level of technology, this might matter more than one might a priori believe.

So, in the end, it seems that anything releasing a dangerous energy effect will only affect a fixed volume. If it has energy $E$ and one can survive it below a deposited energy $e$ , if it just radiates in all directions the safe range is $r = \sqrt{E/4 \pi e} \propto \sqrt{E}$ – one needs to get into supernova ranges to sterilize interstellar volumes. If it is directional the range goes up, but smaller volumes are affected: if a fraction $f$ of the sky is affected, the range increases as $\propto \sqrt{1/f}$ but the total volume affected scales as $\propto f\sqrt{1/f}=\sqrt{f}$ .

Self-sustaining effects are worse, but they need to cross space: if their space range is smaller than interplanetary distances they may destroy a planet but not anything more. For example, a black hole merely absorbs a planet or star (releasing a nasty energy blast) but does not continue sucking up stuff. Vacuum decay on the other hand has indefinite range in space and moves at lightspeed. Accidental self-replication is unlikely to be spaceworthy unless is starts among space-moving machinery; here deliberate design is a more serious problem.

The speed of threat spread also matters. If it is fast enough no escape is possible. However, many of the replicating threats will have sublight speed and could hence be escaped by sufficiently paranoid aliens. The issue here is if lightweight and hence faster replicators can always outrun larger aliens; given the accelerating expansion of the universe it might be possible to outrun them by being early enough, but our calculations do suggest that the margins look very slim.

The more information you have about a target, the better you can in general harm it. If you have no information, merely randomizing it with enough energy/entropy is the only option (and if you have no information of where it is, you need to radiate in all directions). As you learn more, you can focus resources to make more harm per unit expended, up to the extreme limits of solving the optimization problem of finding the informational/environmental inputs that cause desired harm (=hacking). This suggests that mindless threats will nearly always have shorter range and smaller harms than threats designed by (or constituted by) intelligent minds.

In the end, the most likely type of actual civilization-ending threat for an interplanetary civilization looks like it needs to be self-replicating/self-sustaining, able to spread through space, and have at least a tropism towards escaping entities. The smarter, the more effective it can be. This includes both nasty AI and replicators, but also predecessor civilizations that have infrastructure in place. Civilizations cannot be expected to reliably do foolish things with planetary orbits or risky physics.

[Addendum: Charles Stross has written an interesting essay on the risk of griefers as a threat explanation. ]

[Addendum II: Robin Hanson has a response to the rest of us, where he outlines another nasty scenario. ]

Do we want the enhanced military?

Some notes on Practical Ethics inspired by Jonathan D. Moreno’s excellent recent talk.

My basic argument is that enhancing the capabilities of military forces (or any other form of state power) is risky if the probability that they can be misused (or the amount of expected/maximal damage in such cases) does not decrease more strongly. This would likely correspond to some form of moral enhancement, but even the morally enhanced army may act in a bad manner because the values guiding it or the state commanding it are bad: moral enhancement as we normally think about it is all about coordination, the ability to act according to given values and to reflect on these values. But since moral enhancement itself is agnostic about the right values those values will be provided by the state or society. So we need to ensure that states/societies have good values, and that they are able to make their forces implement them. A malicious or stupid head commanding a genius army is truly dangerous. As is tails wagging dogs, or keeping the head unaware (in the name of national security) of what is going on.

In other news: an eclipse in a teacup:

Consequentialist world improvement

I just rediscovered an old response to the Extropians List that might be worth reposting. Slight edits.

On 06/10/2012 16:17, Tomaz Kristan wrote:

>> If you want to reduce death tolls, focus on self-driving cars.
> Instead of answering terror attacks, just mend you cars?

Sounds eminently sensible. Charlie makes a good point: if we want to make the world better, it might be worth prioritizing fixing the stuff that makes it worse according to the damage it actually makes. Toby Ord and me have been chatting quite a bit about this.

Death

In terms of death (~57 million people per year), the big causes are cardiovascular disease (29%), infectious and parasitic diseases (23%) and cancer (12%). At least the first and last are to a sizeable degree caused or worsened by ageing, which is a massive hidden problem. It has been argued that malnutrition is similarly indirectly involved in 15-60% of the total number of deaths: often not the direct cause, but weakening people so they become vulnerable to other risks. Anything that makes a dent in these saves lives on a scale that is simply staggering; any threat to our ability to treat them (like resistance to antibiotics or anthelmintics) is correspondingly bad.

Unintentional injuries are responsible for 6% of deaths, just behind respiratory diseases 6.5%. Road traffic alone is responsible for 2% of all deaths: even 1% safer cars would save 11,400 lives per year. If everybody reached Swedish safety (2.9 deaths per 100,000 people per year) it would save around 460,000 lives per year – one Antwerp per year.

Now, intentional injuries are responsible for 2.8% of all deaths. Of these suicide is responsible for 1.53% of total death rate, violence 0.98% and war 0.3%. Yes, all wars killed about the same number of people as were killed by meningitis, and slightly more than the people who died of syphilis. In terms of absolute numbers we might be much better off improving antibiotic treatments and suicide hotlines than trying to stop the wars. And terrorism is so small that it doesn’t really show up: even the highest estimates put the median fatalities per year in the low thousands.

So in terms of deaths, fixing (or even denting) ageing, malnutrition, infectious diseases and lifestyle causes is a far more important activity than winning wars or stopping terrorists. Hypertension, tobacco, STDs, alcohol, indoor air pollution and sanitation are all far, far more pressing in terms of saving lives. If we had a choice between ending all wars in the world and fixing indoor air pollution the rational choice would be to fix those smoky stoves: they kill nine times more people.

Existential risk

There is of course more to improving the world than just saving lives. First there is the issue of outbreak distributions: most wars are local and small affairs, but some become global. Same thing for pandemic respiratory disease. We actually do need to worry about them more than their median sizes suggest (and again the influenza totally dominates all wars). Incidentally, the exponent for the power law distribution of terrorism is safely strongly negative at -2.5, so it is less of a problem than ordinary wars with exponent -1.41 (where the expectation diverges: wait long enough and you get a war larger than any stated size).

There are reasons to think that existential risk should be weighed extremely strongly: even a tiny risk that we loose all our future is much worse than many standard risks (since the future could be inconceivably grand and involve very large numbers of people). This has convinced me that fixing the safety of governments needs to be boosted a lot: democides have been larger killers than wars in the 20th century and both seems to have most of the tail risk, especially when you start thinking nukes. It is likely a far more pressing problem than climate change, and quite possibly (depending on how you analyse xrisk weighting) beats disease.

How to analyse xrisk, especially future risks, in this kind of framework is a big part of our ongoing research at FHI.

Happiness

If instead of lives lost we look at the impact on human stress and happiness wars (and violence in general) look worse: they traumatize people, and terrorism by its nature is all about causing terror. But again, they happen to a small set of people. So in terms of happiness it might be more important to make the bulk of people happier. Life satisfaction correlates to 0.7 with health and 0.6 with wealth and basic education. Boost those a bit, and it outweighs the horrors of war.

In fact, when looking at the value of better lives, it looks like an enhancement in life quality might be worth much more than fixing a lot of the deaths discussed above: make everybody’s life 1% better, and it corresponds to more quality adjusted life years than is lost to death every year! So improving our wellbeing might actually matter far, far more than many diseases. Maybe we ought to spend more resources on applied hedonism research than trying to cure Alzheimers.

Morality

The real reason people focus so much about terrorism is of course the moral outrage. Somebody is responsible, people are angry and want revenge. Same thing for wars. And the horror tends to strike certain people: my kind of global calculations might make sense on the global scale, but most of us think that the people suffering the worst have a higher priority. While it might make more utilitarian sense to make everybody 1% happier rather than stop the carnage in Syria, I suspect most people would say morality is on the other side (exactly why is a matter of some interesting ethical debate, of course). Deontologists might think we have moral duties we must implement no matter what the cost. I disagree: burning villages in order to save them doesn’t make sense. It makes sense to risk lives in order to save lives, both directly and indirectly (by reducing future conflicts).

But this requires proportionality: going to war in order to avenge X deaths by causing 10X deaths is not going to be sustainable or moral. The total moral weight of one unjust death might be high, but it is finite. Given the typical civilian causality ratio of 10:1 any war will also almost certainly produce far more collateral unjust deaths than the justified deaths of enemy soldiers: avenging X deaths by killing exactly X enemies will still lead to around 10X unjust deaths. So achieving proportionality is very, very hard (and the Just War Doctrine is broken anyway, according to the war ethicists I talk to). This means that if you want to leave the straightforward utilitarian approach and add some moral/outrage weighting, you risk making the problem far worse by your own account. In many cases it might indeed be the moral thing to turn the other cheek… ideally armoured and barbed with suitable sanctions.

Conclusion

To sum up, this approach of just looking at consequences and ignoring who is who is of course a bit too cold for most people. Most people have Tetlockian sacred values and get very riled up if somebody thinks about cost-effectiveness in terrorism fighting (typical US bugaboo) or development (typical warmhearted donor bugaboo) or healthcare (typical European bugaboo). But if we did, we would make the world a far better place.

Bring on the robot cars and happiness pills!

Future Humans: science fiction and human enhancement

Recording of a fun panel we (Imre Bard, Caroline Edwards, Adam Roberts and me) had at the LSE 2015 literary festival about science fiction, human enhancement and cyborgs. Are cyborgs neoliberal, and what about collectivist libertarian solutions like information markets?

Continued integrals

Many of the most awesome formulas you meet when getting into mathematics are continued fractions like

$\Phi = 1+\frac{1}{1+\frac{1}{1+\frac{1}{\ldots}}}$

and nested radicals like

$2 = \sqrt{2 + \sqrt{2 + \sqrt{2 + \ldots}}}$ .

What about nested/continued integrals? Here is a simple one:

$e^x=1+x+\int x+\left(\int x+\left(\int x+\left(\ldots\right)dx\right)dx\right)dx$ .

The way to see this is to recognize that the x in the first integral is going to integrate to $x^2/2$ , the x in the second will be integrated twice $\int x^2/2 dx = x^3/3!$ , and so on.

In general additive integrals of this kind turn into sums (assuming convergence, handwave, handwave…):

$I(x)=\int f(x)+\left(\int f(x)+\left(\int f(x)+\left(\ldots\right)dx\right)dx\right)dx = \sum_{n=1}^\infty \int^n f(x) dx$ .

On the other hand, $I'(x)=f(x)+I(x)$ .

So if we insert $f_k(x)=\sin(kx)$ we get the sum $I_k(x)=-\cos(kx)/k-\sin(kx)/k^2+\cos(kx)/k^3+\sin(x)/k^4-\cos(kx)/k^5-\ldots$ . For $x=0$ we end up with $I_k(0)=\sum_{n=0}^\infty 1/k^{4n+2} - \sum_{n=0}^\infty 2/k^{4n+1}$ . The differential equation has solution $I_k(x)=ce^x-\sin(kx)/(k^2+1) - k\cos(kx)/(k^2+1)$ . Setting $k=0$ the integral is clearly zero, so $c=0$ . Tying it together we get:

$\sum_{n=0}^\infty 1/k^{4n+2}-\sum_{n=0}^\infty 1/k^{4n+1}=-k/(k^2+1)$ .

Things are trickier when the integrals are multiplicative, like $I(x)=\int x \int x \int x \ldots dx dx dx$ . However, we can turn it into a differential equation: $I'(x)=x I(x)$ which has the well known solution $I(x)=ce^{x^2/2}$ . Same thing for $f_k(x)=\sin(kx)$ , giving us $I_k(x)=ce^{-\cos(kx)/k}$ . Since we are running indefinite integrals we get those pesky constants.

Plugging in $f(x)=1/x$ gives $I(x)=cx$ . If we set $c=1$ we get the mildly amusing and in retrospect obvious formula

$x=\int \frac{\int \frac{\int \frac{\ldots}{x} dx}{x} dx}{x} dx$ .

We can of course mess things up further, like $I(x)=\int\sqrt{\int\sqrt{\int\sqrt{\ldots} dx} dx} dx$ , where the differential equation becomes $I'^2=I$ with the solution $I(x)=(1/4)(c^2 + 2cx + x^2)$ . A surprisingly simple solution to a weird-looking integral. In a similar vein:

$2\cot^{-1}(e^{c-x})=\int\sin\left(\int\sin\left(\int\sin\left(\ldots\right)dx\right) dx\right) dx$

$-\log(c-x)=\int \exp\left(\int \exp\left(\int \exp\left(\ldots \right) dx \right) dx \right) dx$

$1/(c-x)=\int \left(\int \left(\int \left(\ldots \right)^2 dx \right)^2 dx \right)^2 dx$

And if you want a real mind-bender, use the Lambert W function:

$I(x)=\int W\left(\int W\left(\int W\left(\ldots \right) dx \right) dx \right) dx$ , then $x=\int_1^{I(x)}1/W(t) dt + c$ .

(that is, you get an implicit but well defined expression for the (x,I(x)) values. With Lambert, the x and y axes always tend to switch place).

[And yes, convergence is handwavy in this essay. I think the best way of approaching it is to view the values of these integrals as the functions invariant under the functional consisting of the integral and its repeated function: whether nearby functions are attracted to it (or not) under repeated application of the functional depends on the case. ]

“A lump of cadmium”

Cadmium crystal and metal. From Wikimedia Commons, Cc: creator Alchemist-hp 2010. — Cadmium crystal and metal. From Wikimedia Commons, creator Alchemist-hp 2010.

Stuart Armstrong sent me this email:

I have a new expression: “a lump of cadmium”.

Background: in WW2, Heisenberg was working on the German atomic reactor project (was he bad? see the fascinating play “Copenhagen” to find out!). His team almost finished a nuclear reactor. He thought that a reaction with natural uranium would be self-limiting (spoiler: it wouldn’t), so had no cadmium control rods or other means of stopping a chain reaction.

But, no worries: his team has “a lump of cadmium” that they could toss into the reactor if things got out of hand. So, now, if someone has a level of precaution woefully inadequate to the risk at hand, I will call it a lump of cadmium.

(Based on German Nuclear Program Before and During World War II by Andrew Wendorff)

It reminds me of the story that SCRAM (emergency nuclear reactor shutdowns) stands for “Safety Control Rod Axe Man“, a guy standing next to the rope suspending the control rods with an axe, ready to cut it. It has been argued it was liquid cadmium solution instead. Still, in the US project they did not assume the reaction was self stabilizing.

Going back to the primary citation, we read:

To understand it we must say something about Heisenberg’s concept of reactor design. He persuaded himself that a reactor designed with natural uranium and, say, a heavy water moderator would be self-stabilizing and could not run away. He noted that U(238) has absorption resonances in the 1-eV region, which means that a neutron with this kind of energy has a good chance of being absorbed and thus removed from the chain reaction. This is one of the challenges in reactor design—slowing the neutrons with the moderator without losing them all to absorption. Conversely, if the reactor begins to run away (become supercritical) , these resonances would broaden and neutrons would be more readily absorbed. Moreover, the expanding material would lengthen the mean free paths by decreasing the density and this expansion would also stop the chain reaction. In short, we might experience a nasty chemical explosion but not a nuclear holocaust. Whether Heisenberg realized the consequences of such a chemical explosion is not clear. In any event, no safety elements like cadmium rods were built into Heisenberg’s reactors. At best, a lump of cadmium was kepton hand in case things threatened to get out of control. He also never considered delayed neutrons, which, as we know, play an essential role in reactor safety. Because none of Heisenberg’s reactors went critical, this dubious strategy was never put to the test.

(Jeremy Bernstein, Heisenberg and the critical mass. Am. J. Phys. 70, 911 (2002); http://dx.doi.org/10.1119/1.1495409)

This reminds me a lot of the modelling errors we discuss in the “Probing the improbable” paper, especially of course the (ahem) energetic error giving Castle Bravo 15 megatons of yield instead of the predicted 4-8 megatons. Leaving out Li(7) from the calculations turned out to leave out the major contributor of energy.

Note that Heisenberg did have an argument for his safety, in fact two independent ones! The problem might have been that he was thinking in terms of mostly U(238) and then getting any kind of chain reaction going would be hard, so he was biased against the model of explosive chain reactions (but as the Bernstein paper notes, somebody in the project had correct calculations for explosive critical masses). Both arguments were flawed when dealing with reactors enriched in U(235). Coming at nuclear power from the perspective of nuclear explosions on the other hand makes it natural to consider how to keep things from blowing up.

We may hence end up with lumps of cadmium because we approach a risk from the wrong perspective. The antidote should always be to consider the risks from multiple angles, ideally a few adversarial ones. The more energy, speed or transformative power we expect something to produce, the more we should scrutinize existing safeguards for them being lumps of cadmium. If we think our project does not have that kind of power, we should both question why we are even doing it, and whether it might actually have some hidden critical mass.

The 12 threats of xrisk

The Global Challenges Foundation has (together with FHI) produced a report on the 12 risks that threaten civilization.

And, yes, the use of “infinite impact” grates on me – it must be interepreted as “so bad that it is never acceptable”, a ruin probability, or something similar, not that the disvalue diverges. But the overall report is a great start on comparing and analysing the big risks. It is worth comparing it with the WEF global risk report, which focuses on people’s perceptions of risk. This one aims at looking at what risks are most likely/impactful. Both try to give reasons and ideas for how to reduce the risks. Hopefully they will also motivate others to make even sharper analysis – this is a first sketch of the domain, rather than a perfect roadmap. Given the importance of the issues, it is a bit worrying that it has taken us this long.

Gamma function surfaces

The gamma function has a long and interesting history (check out (Davis 1963) excellent review), but one application does not seem to have shown up: minimal surfaces.

A minimal surface is one where the average curvature is always zero; it bends equally in two opposite directions. This is equivalent to having the (locally) minimal area given its boundary: such surfaces are commonly seen as soap films stretched from frames. There exists a rich theory for them, linking them to complex analysis through the Enneper-Weierstrass representation: if you have a meromorphic function g and an analytic function f such that $fg^2$ is holomorphic, then

$X(z)=\Re\left(\int_{z_0}^z f(1-g^2)/2 dz\right)$
$Y(z)=\Re\left(\int_{z_0}^z if(1+g^2)/2 dz\right)$
$Z(z)=\Re\left(\int_{z_0}^z fg dz\right)$

produces a minimal surface $(X(z),Y(z),Z(z))$ .

When plugging in the hyperbolic tangent as g and using f=1 I got a new and rather nifty surface a few years back. What about plugging in the gamma function? Let $f=1, g=\Gamma(z)$ .

We integrate from the regular point $z_0=1$ to different points $z$ in the complex plane. Let us start with the simple case of $\Re(z)>1/2$ .

Gamma function minimal surface for z in 0.5<Re(z)<3.5, -8<Im(z)<8. Color denotes Re(z). — Gamma function minimal surface for z in 0.5<Re(z)<3.5, -8<Im(z)

The surface is a billowing strip, and as we include z with larger and larger real parts the amplitude of the oscillations grow rapidly, making it self-intersect. The behaviour is somewhat similar to the Catalan minimal surface, except that we only get one period. If we go to larger imaginary parts the surface approaches a horizontal plane. OK, the surface is a plane with some wild waves, right?

Not so fast, we have not looked at the mess for Re(z)<0. First, let’s examine the area around the z=0 singularity. Since the values of the integrand blows up close to it, they produce a surface expanding towards infinity – very similar to a catenoid. Indeed, catenoid ends tend to show up where there are poles. But this one doesn’t close exactly: for re(z)<0 there is some overshoot producing a self-intersecting plane-like strip.

Gamma function minimal surface close to the z=0 singularity. Colour denotes Re(z). Integration contours from 1 to z run clockwise for Im(z)0. — Gamma function minimal surface close to the z=0 singularity. Colour denotes Re(z). Integration contours from 1 to z run clockwise for Im(z)<0 and counterclockwise for Im(z)>0.

The problem is of course the singularity: when integrating in the complex plane we need to avoid them, and depending on the direction we go around them we can get a complex phase that gives us an entirely different value of the function. In this case the branch cut corresponds to the real line: integrating clockwise or counter-clockwise around z=0 to the same z gives different values. In fact, a clockwise turn adds [3.6268i, 3.6268, 6.2832i] (which looks like $\gamma\pi$ – a rather neat residue!) to the coordinates: a translation in the positive y-direction. If we extend the surface by going an extra turn clockwise or counterclockwise a number of times, we get copies that attach seamlessly.

Gamma minimal surface extended by integration paths between the -1 and 0 singularities (blue patches).

Gamma minimal surface patch that can be repeated by translation along the y-axis. Colour denotes Re(z).

OK, we have a surface with some planar strips that turn wobbly and self-intersecting in the x-direction, with elliptic catenoid ends repeating along the y-direction due to the z=0 singularity. Going down the negative x-direction things look plane between the catenoids… except of course for the catenoids due to all the other singularities for $z=-1,-2,\ldots$ . They also introduce residues along the y-direction, but different ones from the z=0 – their extensions of the surface will be out of phase with each other, making the fully extended surface fantastically self-intersecting and confusing.

Gamma function minimal surface extended by integrating around poles.

So, I think we have a simple answer to why the gamma function minimal surface is not well known: it is simply too messy and self-intersecting.

Of course, there may be related nifty surfaces. $1/\Gamma(z)$ is nicely behaved and looks very much like the Enneper surface near zero, with “wings” that oscillate ever more wildly as we move towards the negative reals. No doubt there are other beautiful things to look for in the vicinity.

Canine mechanics and banking

There are some texts that are worth reading, even if you are outside the group they are intended for. Here is one that I think everybody should read at least the first half of:

Andrew G Haldane and Vasileios Madouros: The dog and the frisbee

Haldane, the Executive Director for Financial Stability at Bank of England, brings up the topic is how to act in situations of uncertainty, and the role of our models of reality in making the right decision. How complex should they be in the face of a complex reality? The answer, based on the literature on heuristics, biases and modelling, and the practical world of financial disasters, is simple: they should be simple.

Using too complex models means that they tend to overfit scarce data, weight data randomly, require significant effort to set up – and tends to promote overconfidence. As Haldane then moves on to his own main topic, banking regulation. Complex regulations – which are in a sense models of how banks ought to act – have the same problem, and also act as incentives for playing the rules to gain advantage. The end result is an enormous waste of everybody’s time and effort that does not give the desired reduction of banking risk.

It is striking how many people have been seduced by the siren call of complex regulation or models, thinking their ability to include every conceivable special case is a sign of strength. Finance and finance regulation are full of smart people who make the same mistake, as is science. If there is one thing I learned in computational biology is that your model better produce more nontrivial results than the number of parameters it has.

But coming up with simple rules or models is not easy: knowing what to include and what not to include requires expertise and effort. In many ways this may be why people like complex models, since there are no tricky judgement calls.

Gamma function fractals

Another of my favourite functions if the Gamma function, $\Gamma(z)=\int_0^\infty t^{z-1}e^{-t} dt$ , the continuous generalization of the factorial. While it grows rapidly for positive reals, it has fun poles for the negative integers and is generally complex. What happens when you iterate it?

First I started by just applying it to different starting points, $z_{n+1} = \Gamma(z_n)$ . The result is a nice fractal, with some domains approaching 1, and others running off to infinity.

Here I color points that go to infinity in green shades on the number of iterations before they become very large, and the points approaching 1 by $|z_{30}-1|$ . Zooming in a bit more reveals neat self-similar patterns with alternating “beans”:

In the outside regions we have thin tendrils stretching towards infinity. These are familiar to anybody who has been iterating exponentials or trigonometric functions: the combination of oscillation and (super)exponential growth leads to the pattern.

OK,that was a Julia set (different starting points, same formula). What about a counterpart to the Mandelbrot set? I looked at $z_{n+1}=\Gamma(cz_n)$ where c is the control parameter. I start with $z_0=1$ and iterate:

Zooming in shows the same kind of motif copies of Julia sets as we see in the quadratic Mandelbrot set:

In fact, zooming in as above in the counterpart to the “seahorse valley” shows a remarkable similarity.

Andart II

Part of Anders' Exoself

Author: admin