It is Newtonmass, and that means doing some fun math. I try to invent a new fractal or something similar every year: this is the result for 2018.
The Newton fractal is an old classic. Newton’s method for root finding iterates an initial guess to find a better approximation
. This will work as long as
, but which root one converges to can be sensitively dependent on initial conditions. Plotting which root a given initial value ends up with gives the Newton fractal.
The Newton-Gauss method is a method for minimizing the total squared residuals when some function dependent on n-dimensional parameters $\beta$ is fitted to
data points
. Just like the root finding method it iterates towards a minimum of
:
where
is the Jacobian
. This is essentially Newton’s method but in a multi-dimensional form.
So we can make fractals by trying to fit (say) a function consisting of the sum of two Gaussians with different means (but fixed variances) to a random set of points. So we can set (one Gaussian with variance 1 and one with 1/4 – the reason for this is not to make the diagram boringly symmetric as for the same variance case). Plotting the location of the final
(by stereographically mapping it onto a unit sphere in (r,g,b) space) gives a nice fractal:
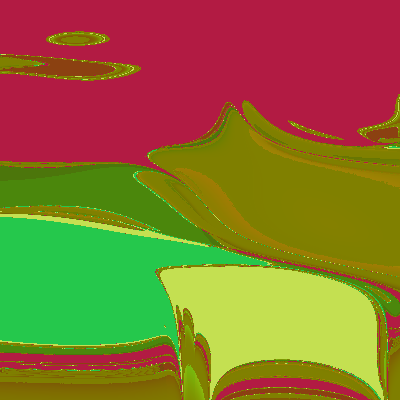
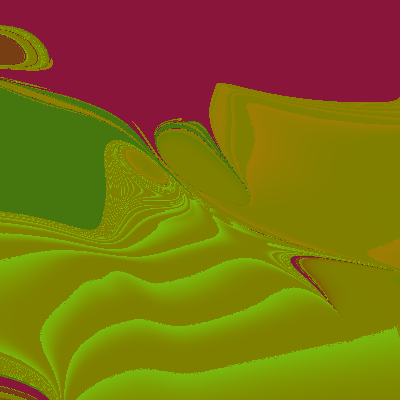
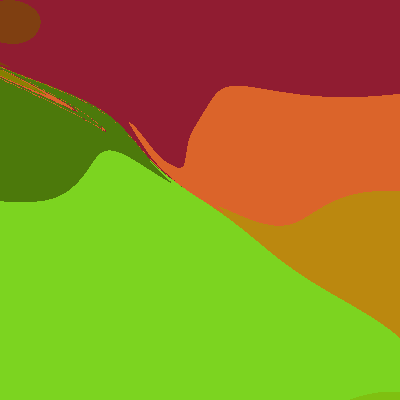
It is a bit modernistic-looking. As I argued in 2016, this is because the generic local Jacobian of the dynamics doesn’t have much rotation.
As more and more points are added the attractor landscape becomes simpler, since it is hard for the Gaussians to “stick” to some particular clump of points and the gradients become steeper.
This fractal can obviously be generalized to more dimensions by using more parameters for the Gaussians, or more Gaussians etc.
The fractality is guaranteed by the generic property of systems with several attractors that points at the border of two basins of attraction will tend to find their ways to other attractors than the two equally balanced neighbors. Hence a cut transversally across the border will find a Cantor-set of true basin boundary points (corresponding to points that eventually get mapped to a singular Jacobian in the iteration formula, like the boundary of the Newton fractal is marked by points mapped to for some n) with different basins alternating.
Merry Newtonmass!