Month: December 2016

Newtonmas fractals: conquering the second dimension!

Perturbed Newton "classic", epsilon=-3.75.
Perturbed Newton “classic”, epsilon=-3.75.

It is Newtonmas, so time to invent some new fractals.

Complex iteration of Newton’s method for finding zeros of a function is a well-known way of getting lovely filigree Julia sets: the basins of attraction of the different zeros have fractal borders.

But what if we looked at real functions? If we use a single function f(x,y) the zeros will typically form a curve in the plane. In order to get discrete zeros we typically need to have two functions to produce a zero set. We can think of it as a map from R2 to R2 F(x)=[f_1(x_1,x_2), f_2(x_1,x_2)] where the x’es are 2D vectors. In this case Newton’s method turns into solving the linear equation system J(x_n)(x_{n+1}-x_n)=-F(x_n) where J(x_n) is the Jacobian matrix (J_{ij}=dF_i/dx_j) and x_n now denotes the n’th iterate.

The simplest case of nontrivial dynamics of the method is for third degree polynomials, and we want the x- and y-directions to interact a bit, so a first try is F=[x^3-x-y, y^3-x-y]. Below is a plot of the first and second components (red and green), as well as a blue plane for zero values. The zeros of the function are the three points where red, green and blue meet.

We have three zeros, one at x=y=-\sqrt{2}, one at x=y=0, and one at x=y=\sqrt{2}. The middle one has a region of troublesomely similar function values – the red and green surfaces are tangent there.

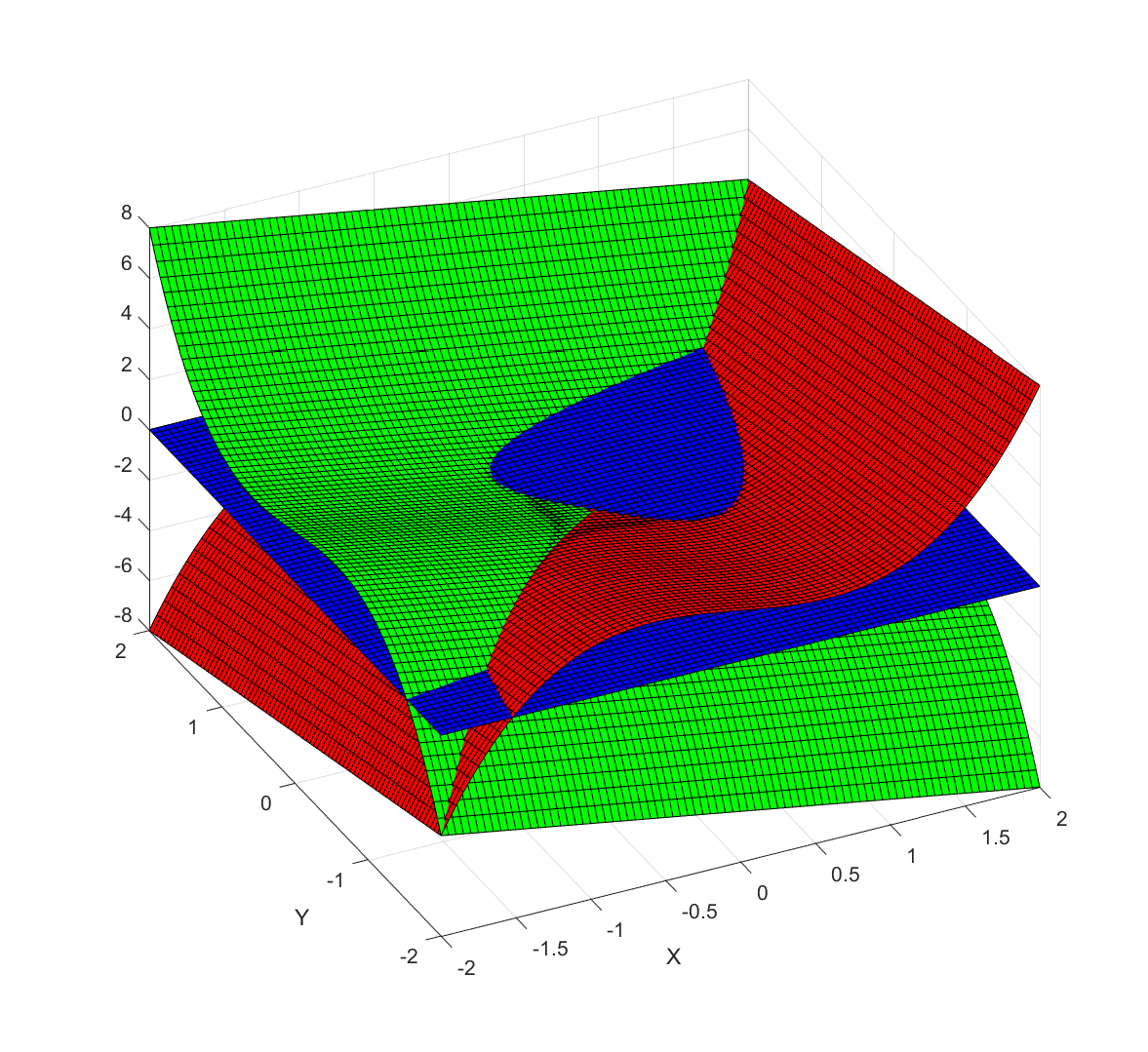
Plot of x^3-x-y (red), y^3-x-y (green) and z=0 (blue). The zeros found using Newton's method are the points where red, green and blue meet.
Plot of x^3-x-y (red), y^3-x-y (green) and z=0 (blue). The zeros found using Newton’s method are the points where red, green and blue meet.

The resulting fractal has a decided modernist bent to it, all hyperbolae and swooshes:

Behavior of Newton's method in 2D for F=[x^3-x-y, y^3-x-y]. Color denotes value of x+y, with darkening for slow convergence.
Behavior of Newton’s method in 2D for F=[x^3-x-y, y^3-x-y]. Color denotes value of x+y, with darkening for slow convergence.
The troublesome region shows up, as well as the red and blue regions where iterates run off to large or small values: the three roots are green shades.

Why is the style modernist?

In complex iterations you typically multiply with complex numbers, and if they have an imaginary component (they better have, to be complex!) that introduces a rotation or twist. Hence baroque filaments are ubiquitous, and you get the typical complex “style”.

But here we are essentially multiplying with a real matrix. For a real 2×2 matrix to be a rotation matrix it has to have a pair of imaginary eigenvalues, and for it to at least twist things around the trace needs to be small enough compared to the determinant so that there are complex eigenvalues: T^2/4<D (where T=a+d and D=ad-bc if the matrix has the usual [a b; c d] form). So if the trace and determinant are randomly chosen, we should expect a majority of cases to be non-rotational.

Moreover, in this particular case, the Jacobian tends to be diagonally dominant (quadratic terms on the diagonal) and that makes the inverse diagonally dominant too: the trace will be big, and hence the chance of seeing rotation goes down even more. The two “knots” where a lot of basins of attraction come together are the points where the trace does vanish, but since the Jacobian is also symmetric there will not be any rotation anyway. Double guarantee.

Can we make a twisty real Newton fractal? If we start with a vanilla rotation matrix and try to find a function that produces it the simplest case is F=[x \cos(\theta) + y \sin(\theta), x\sin(\theta)+y\cos(\theta)]. This is of course just a rotation by the angle theta, and it does not have very interesting zeros.

To get something fractal we need more zeros, and a few zeros in the derivatives too (why? because they cause iterates to be thrown away far from where they were, ensuring a complicated structure of the basin boundaries). One attempt is F=[ (x^3-x-y) \cos(\theta) -(y^3-x-y) \sin(\theta), (x^3-x-y) \sin(\theta)+(y^3-y-x) \cos(\theta) ]. The result is fun, but still far from baroque:

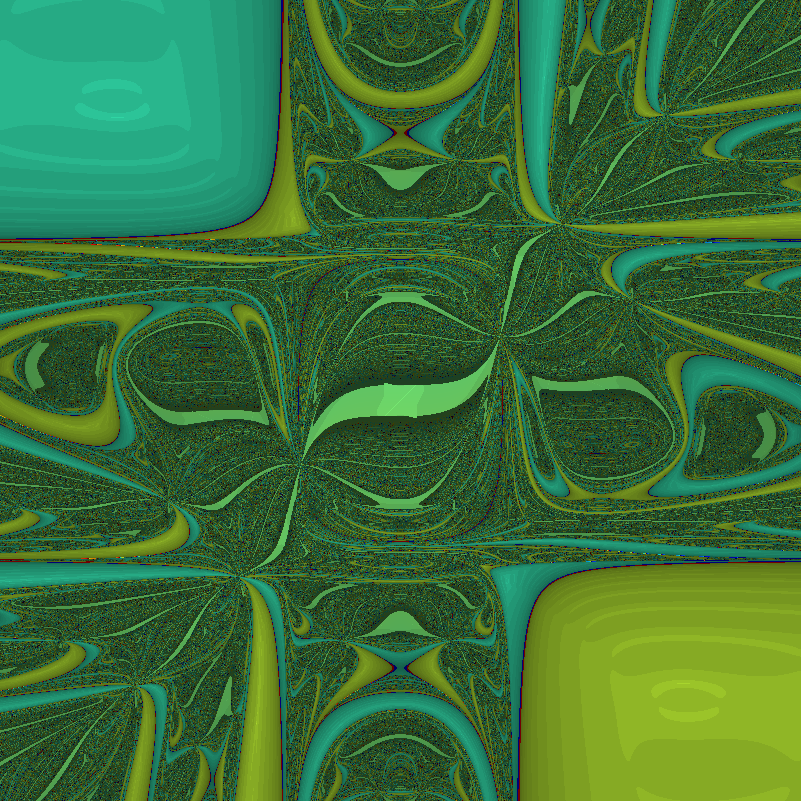
Basins of attraction for Netwon's method of twisted cubic. theta=1.
Basins of attraction for Netwon’s method of twisted cubic. theta=1.
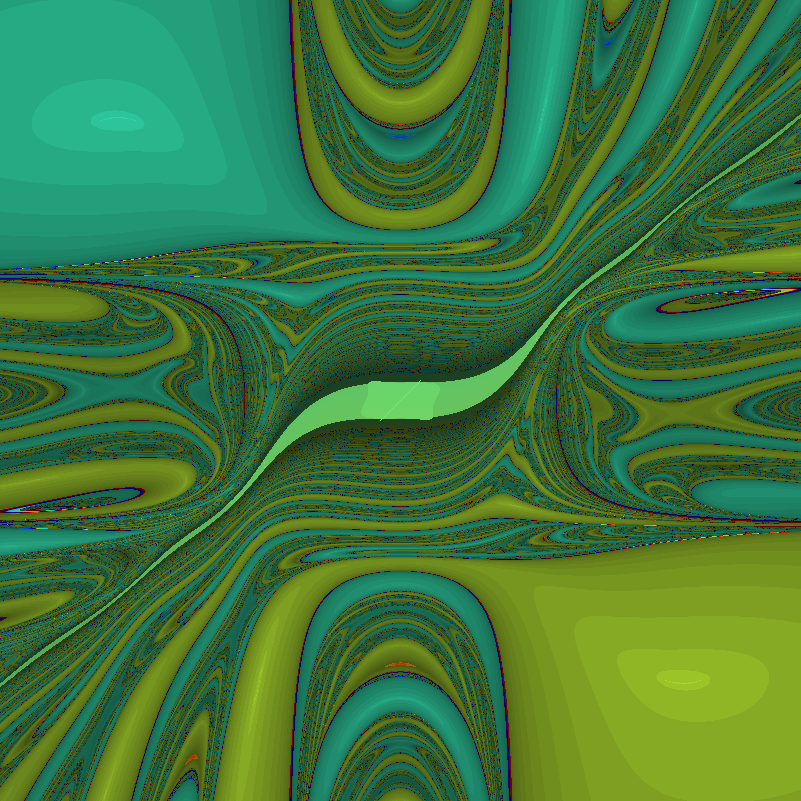
Basins of attraction for Netwon's method of twisted cubic. theta=0.1.
Basins of attraction for Netwon’s method of twisted cubic. theta=0.1.

The problem might be that the twistiness is not changing. So we can make \theta=x to make the dynamics even more complex:

Basins of attraction with rotation proportional to x.
Basins of attraction with rotation proportional to x.

Quite lovely, although still not exactly what I wanted (sounds like a Christmas present).

Back to the classics?

It might be easier just to hide the complex dynamics in an apparently real function like F=[x^3-3xy^2-1, 3x^2y-y^3] (which produces the archetypal f(z)=z^3-1 Newton fractal).

Newton fractal for F=[x^3-3xy^2-1, 3x^2y-y^3].
Newton fractal for F=[x^3-3xy^2-1, 3x^2y-y^3]. Red and blue circles mark regions where iterates venture far from the origin.
It is interesting to see how much perturbing it causes a modernist shift. If we use F=[x^3-3xy^2-1 + \epsilon x, 3x^2y-y^3], then for \epsilon=1 we get:

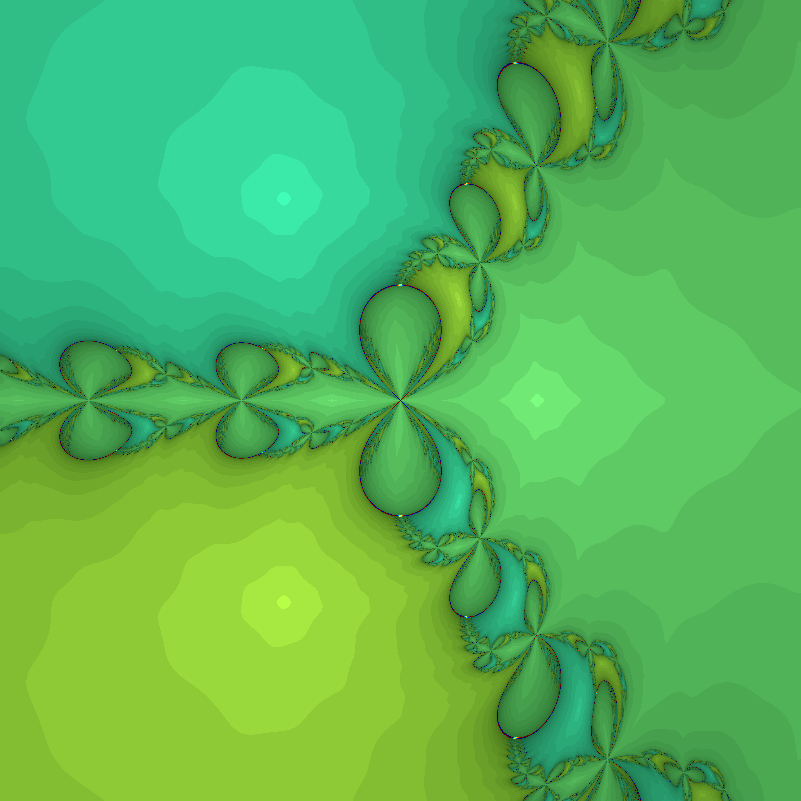
Perturbed z^3-1 Newton iteration, epsilon=1.
Perturbed z^3-1 Newton iteration, epsilon=1.

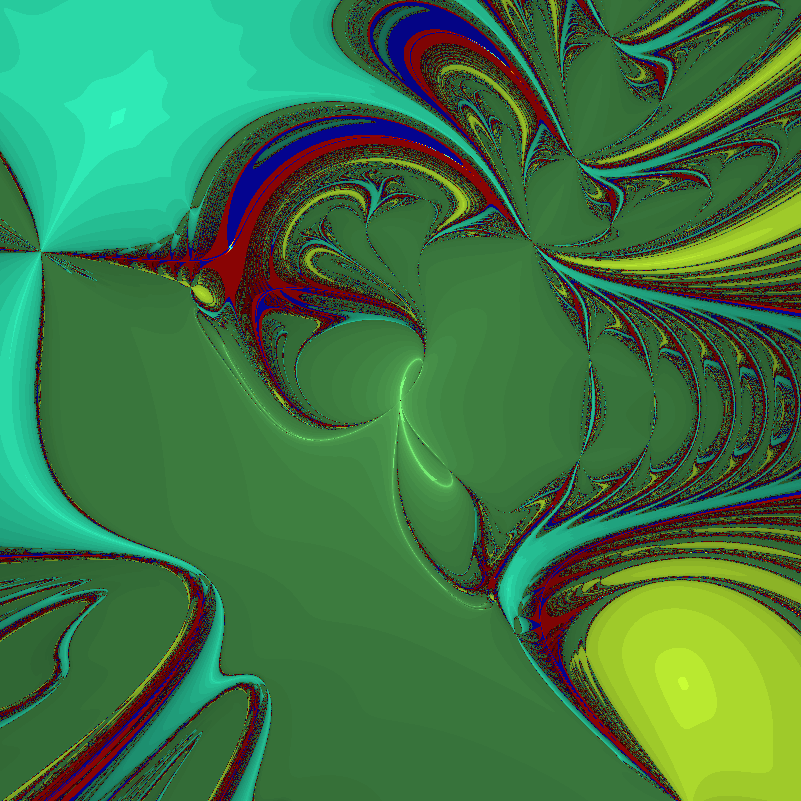
As we make the function more perturbed, it turns more modernist, undergoing a topological crisis for epsilon between 3.5 and 4:

Perturbed z^3-1 Newton iteration, epsilon=2.
Perturbed z^3-1 Newton iteration, epsilon=2.
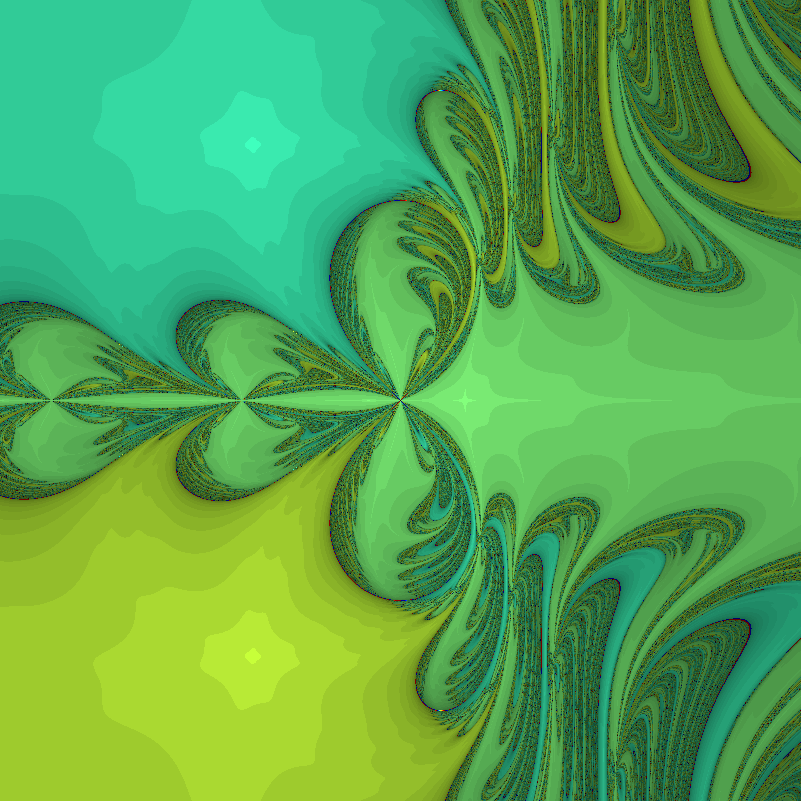
Perturbed z^3-1 Newton iteration, epsilon=3.
Perturbed z^3-1 Newton iteration, epsilon=3.
Perturbed z^3-1 Newton iteration, epsilon=3.5.
Perturbed z^3-1 Newton iteration, epsilon=3.5.
Perturbed z^3-1 Newton iteration, epsilon=4.
Perturbed z^3-1 Newton iteration, epsilon=4.

In the end, we can see that the border between classic baroque complex fractals and the modernist swooshy real fractals is fuzzy. Or, indeed, fractal.

A crazy futurist writes about crazy futurists

Arjen the doomsayerWarren Ellis’ Normal is a little story about the problem of being serious about the future.

As I often point out, most people in the futures game are basically in the entertainment industry: telling wonderful or frightening stories that allow us to feel part of a bigger sweep of history, reflect a bit, and then return to the present with the reassurance that we have some foresight. Relatively little future studies is about finding decision-relevant insights and then acting on it. It exists, but it is not the bulk of future-oriented people. Taking the future seriously might require colliding with your society as you try to tell it it is going the wrong way. Worse, the conclusions might tell you that your own values and goals are wrong.

Normal takes place at a sanatorium for mad futurists in the wilds of Oregon. The idea is that if you spend too much time thinking too seriously about the big and horrifying things in the future mental illness sets in. So when futurists have nervous breakdowns they get sent by their sponsors to Normal to recover. They are useful, smart, and dedicated people but since the problems they deal with are so strange their conditions are equally unusual. The protagonist arrives just in time to encounter a bizarre locked room mystery – exactly the worst kind of thing for a place like Normal with many smart and fragile minds – driving him to investigate what is going on.

As somebody working with the future, I think the caricatures of these futurists (or rather their ideas) are spot on. There are the urbanists, the singularitarians, the neoreactionaries, the drone spooks, and the invented professional divisions. Of course, here they are mad in a way that doesn’t allow them to function in society which softballs the views: singletons and Molochs are serious real ideas that should make your stomach lurch.

The real people I know who take the future seriously are overall pretty sane. I remember a documentary filmmaker at a recent existential risk conference mildly complaining that people where so cheerful and well-adapted: doubtless some darkness and despair would have made a far more compelling imagery than chummy academics trying to salvage the bioweapons convention. Even the people involved in developing the Mutually Assured Destruction doctrine seem to have been pretty healthy. People who go off on the deep end tend to do it not because of The Future but because of more normal psychological fault lines. Maybe we are not taking the future seriously enough, but I suspect it is more a case of an illusion of control: we know we are at least doing something.

This book convinced me that I need to seriously start working on my own book project, the “glass is half full” book. Much of our research at FHI seems to be relentlessly gloomy: existential risk, AI risk, all sorts of unsettling changes to the human condition that might slurp us down into a valueless attractor asymptoting towards the end of time. But that is only part of it: there are potential futures so bright that we do not just need sunshades, but we have problems with even managing the positive magnitude in an intellectually useful way. The reason we work on existential risk is that we (1) think there is enormous positive potential value at stake, and (2) we think actions can meaningfully improve chances. That is no pessimism, quite the opposite. I can imagine Ellis or one of his characters skeptically looking at me across the table at Normal and accusing me of solutionism and/or a manic episode. Fine. I should lay out my case in due time, with enough logos, ethos and pathos to convince them (Muhahaha!).

I think the fundamental horror at the core of Normal – and yes, I regard this more as a horror story than a techno-thriller or satire – is the belief that The Future is (1) pretty horrifying and (2) unstoppable. I think this is a great conceit for a story and a sometimes necessary intellectual tonic to consider. But it is bad advice for how to live a functioning life or actually make a saner future.

 

Settling Titan, Schneier’s Law, and scenario thinking

Charles Wohlforth and Amanda R. Hendrix want us to colonize Titan. The essay irritated me in an interesting manner.

Full disclosure: they interviewed me while they were writing their book Beyond Earth: Our Path to a New Home in the Planets, which I have not read yet, and I will only be basing the following on the SciAm essay. It is not really about settling Titan either, but something that bothers me with a lot of scenario-making.

A weak case for Titan and against Luna and Mars

titan2dmapBasically the essay outlines reasons why other locations in the solar system are not good: Mercury too hot, Venus way too hot, Mars and Luna have too much radiation. Only Titan remains, with a cold environment but not too much radiation.

A lot of course hinges on the assumptions:

We expect human nature to stay the same. Human beings of the future will have the same drives and needs we have now. Practically speaking, their home must have abundant energy, livable temperatures and protection from the rigors of space, including cosmic radiation, which new research suggests is unavoidably dangerous for biological beings like us.

I am not that confident in that we will remain biological or vulnerable to radiation. But even if we decide to accept the assumptions, the case against the Moon and Mars is odd:

Practically, a Moon or Mars settlement would have to be built underground to be safe from this radiation.Underground shelter is hard to build and not flexible or easy to expand. Settlers would need enormous excavations for room to supply all their needs for food, manufacturing and daily life.

So making underground shelters is much harder than settling Titan, where buildings need to be isolated against a -179 C atmosphere and ice ground full with complex and quite likely toxic hydrocarbons. They suggest that there is no point in going to the moon to live in an underground shelter when you can do it on Earth, which is not too unreasonable – but is there a point in going to live inside an insulated environment on Titan either? The actual motivations would likely be less of a desire for outdoor activities and more scientific exploration, reducing existential risk, and maybe industrialization.

Also, while making underground shelters in space may be hard, it does not look like an insurmountable problem. The whole concern is a bit like saying submarines are not practical because the cold of the depths of the ocean will give the crew hypothermia – true, unless you add heating.

I think this is similar to Schneier’s law:

Anyone, from the most clueless amateur to the best cryptographer, can create an algorithm that he himself can’t break.

It is not hard to find a major problem with a possible plan that you cannot see a reasonable way around. That doesn’t mean there isn’t one.

Settling for scenarios

9 of Matter: The Planet GardenMaybe Wohlforth and Hendrix spent a lot of time thinking about lunar excavation issues and consistent motivations for settlements to reach a really solid conclusion, but I suspect that they came to the conclusion relatively lightly. It produces an interesting scenario: Titan is not the standard target when we discuss where humanity ought to go, and it is an awesome environment.

Similarly the “humans will be humans” scenario assumptions were presumably chosen not after a careful analysis of relative likelihood of biological and postbiological futures, but just because it is similar to the past and makes an interesting scenario. Plus human readers like reading about humans rather than robots. All together it makes for a good book.

Clearly I have different priors compared to them on the ease and rationality of Lunar/Martian excavation and postbiology. Or even giving us D. radiodurans genes.

In The Age of Em Robin Hanson argues that if we get the brain emulation scenario space settlement will be delayed until things get really weird: while postbiological astronauts are very adaptable, so much of the mainstream of civilization will be turning inward towards a few dense centers (for economics and communications reasons). Eventually resource demand, curiosity or just whatever comes after the Age of Ems may lead to settling the solar system. But that process will be pretty different even if it is done by mentally human-like beings that do need energy and protection. Their ideal environments would be energy-gradient rich, with short communications lags: Mercury, slowly getting disassembled into a hot Dyson shell, might be ideal. So here the story will be no settlement, and then wildly exotic settlement that doesn’t care much about the scenery.

But even with biological humans we can imagine radically different space settlement scenarios, such as the Gerhard O’Neill scenario where planetary surfaces are largely sidestepped for asteroids and space habitats. This is Jeff Bezo’s vision rather than Elon Musk’s and Wohlforth/Hendrix’s. It also doesn’t tell the same kind of story: here our new home is not in the planets but between them.

My gripe is not against settling Titan, or even thinking it is the best target because of some reasons. It is against settling too easily for nice scenarios.

Beyond the good story

Sometimes we settle for scenarios because they tell a good story. Sometimes because they are amenable to study among other, much less analyzable possibilities. But ideally we should aim at scenarios that inform us in a useful way about options and pathways we have.

That includes making assumptions wide enough to cover relevant options, even the less glamorous or tractable ones.

That requires assuming future people will be just as capable (or more) at solving problems: just because I can’t see a solution to X doesn’t mean it is not trivially solved in the future.

(Maybe we could call it the “Manure Principle” after the canonical example of horse manure being seen as a insoluble urban planning problem at the previous turn of century and then neatly getting resolved by unpredicted trams and cars – and just like Schneier’s law and Stigler’s law the reality is of course more complex than the story.)

In standard scenario literature there are often admonitions not just to select a “best case scenario”, “worst case scenario” and “business as usual scenario” – scenario planning comes into its own when you see nontrivial, mixed value possibilities. In particular, we want decision-relevant scenarios that make us change what we will do when we hear about them (rather than good stories, which entertain but do not change our actions). But scenarios on their own do not tell us how to make these decisions: they need to be built from our rationality and decision theory applied to their contents. Easy scenarios make it trivial to choose (cake or death?), but those choices would have been obvious even without the scenarios: no forethought needed except to bring up the question. Complex scenarios force us to think in new ways about relevant trade-offs.

The likelihood of complex scenarios is of course lower than simple scenarios (the conjunction fallacy makes us believe much more in rich stories). But if they are seen as tools for developing decisions rather than information about the future, then their individual probability is less of an issue.

In the end, good stories are lovely and worth having, but for thinking and deciding carefully we should not settle for just good stories or the scenarios that feel neat.